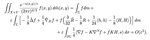François-Xavier Vialard
Enseignant-chercheur au Laboratoire d’informatique Gaspard Monge.
Interests
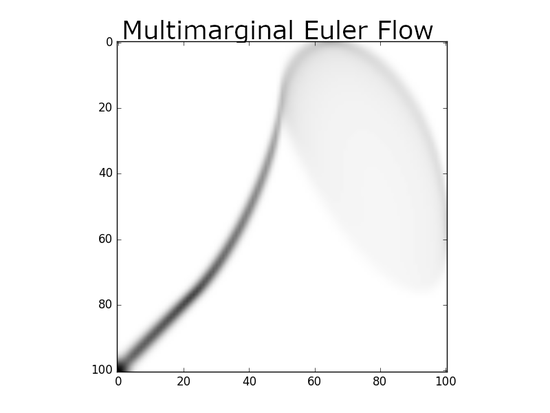
- Optimal transport; applications and numerics.
- Diffeomorphic flows; machine learning and medical image registration applications.
- Calculus of variations and geometry; applications to shape spaces and fluid flows.
- Applied and computational mathematics.