Entropic Semi-Martingale Optimal Transport

Abstract

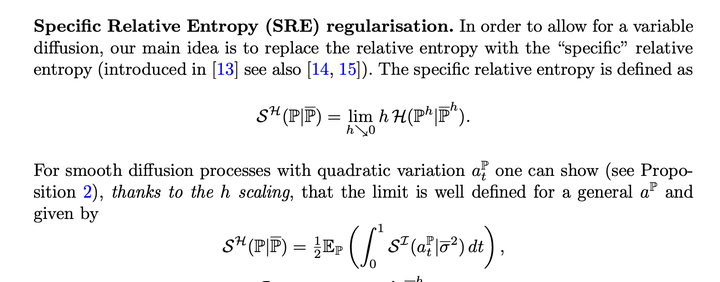
Entropic Optimal Transport (EOT), also referred to as the Schrödinger problem, seeks to find a random processes with prescribed initial/final marginals and with minimal relative entropy with respect to a reference measure. The relative entropy forces the two measures to share the same support and only the drift of the controlled process can be adjusted, the diffusion being imposed by the reference measure. Therefore, at first sight, Semi-Martingale Optimal Transport (SMOT) problems (see [1]) seem out of the scope of applications of Entropic regularization techniques, which are otherwise very attractive from a computational point of view. However, when the process is observed only at discrete times, and become therefore a Markov chain, its relative entropy can remain finite even with variable diffusion coefficients, and discrete semi-martingales can be obtained as solutions of (multi-marginal) EOT problems.Given a (smooth) semi-martingale, the limit of the relative entropy of its time discretizations, scaled by the time step converges to the so-called ``specific relative entropy”, a convex functional of its variance process, similar to those used in this http URL this paper we use this observation to build an entropic time discretization of continuous SMOT problems. This allows to compute discrete approximations of solutions to continuous SMOT problems by a multi-marginal Sinkhorn algorithm, without the need of solving the non-linear Hamilton-Jacobi-Bellman pde’s associated to the dual problem, as done for example in [1, 2]. We prove a convergence result of the time discrete entropic problem to the continuous time problem, we propose an implementation and provide numerical experiments supporting the theoretical convergence.